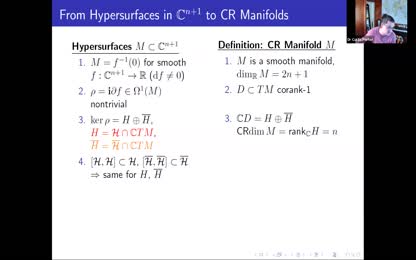
Curtis Porter : CRash CouRse in CR Geometry
- Graduate/Faculty Seminar,Uploaded Videos ( 2171 Views )CR geometry studies real hypersurfaces in complex vector spaces and their generalizations, CR manifolds. In many cases of interest to complex analysis and PDE, CR manifolds can be considered ``curved versions" of homogeneous spaces according to Elie Cartan’s generalization of Klein’s Erlangen program. Which homogeneous space is the ``flat model" of a CR manifold depends on the Levi form, a tensor named after a mathematician who used it to characterize boundaries of pseudoconvex domains. As in the analytic setting, the Levi form plays a central role in the geometry of CR manifolds, which we explore in relation to their homogeneous models.
Holden Lee : Recovering sparse Fourier signals, with application to system identification
- Graduate/Faculty Seminar,Uploaded Videos ( 1018 Views )The problem of recovering a sparse Fourier signal from samples comes up in signal processing, imaging, NMR spectroscopy, and machine learning. Two major challenges involve dealing with off-grid frequencies, and dealing with signals lacking separation between frequencies. Without a minimum separation condition, the problem of frequency recovery is exponentially ill-conditioned, but the signal can still be efficiently recovered in an "improper" manner using an appropriate filter. I will explain such an algorithm for sparse Fourier recovery, and the theory behind why it works - involving some clever analytic inequalities for Fourier-sparse signals. Finally, I will discuss recent work with Xue Chen on applying these ideas to system identification. Identification of a linear dynamical system from partial observations is a fundamental problem in control theory. A natural question is how to do so with statistical rates depending on the inherent dimensionality (or order) of the system, akin to the sparsity of a signal. We solve this question by casting system identification as a "multi-scale" sparse Fourier recovery problem.
Stochastic and continuum dynamics in intracellular transport
- Graduate/Faculty Seminar,Uploaded Videos ( 1071 Views )The cellular cytoskeleton is made up of protein polymers (filaments) that are essential in proper cell and neuronal function as well as in development. These filaments represent the roads along which most protein transport occurs inside cells. I will discuss several examples where questions about filament-cargo interactions require the development of novel mathematical modeling, analysis, and simulation. Protein cargoes such as neurofilaments and RNA molecules bind to and unbind from cellular roads called microtubules, switching between bidirectional transport, diffusion, and stationary states. Since these transport models can be analytically intractable, we have proposed asymptotic methods in the framework of partial differential equations and stochastic processes which are useful in understanding large-time transport properties. I will discuss a recent project where we use stochastic modeling to understand how filament orientations may influence sorting of cargo in dendrites during neural development and axonal injury.
Yiming Zhong : Fast algorithm for Radiative transport
- Graduate/Faculty Seminar,Uploaded Videos ( 944 Views )This talk consists of two aspects about solving the radiative transport through the integral formulation. The radiative transport equation has been numerically studied for many years, the equation is difficult to solve due to its high dimensionality and its hyperbolic nature, in recent decades, the computers are equipped with larger memories so it is possible to deal with the full-discretization in phase space, however, the numerical efficiency is quite limited because of many issues, such as iterative scheme, preconditioning, discretization, etc. In this talk, we first discuss about the special case of isotropic scattering and its integral formulation, then walk through the corresponding fast algorithm for it. In the second part, we try to trivially extend the method to anisotropic case, and talk about the method’s limitation and some perspectives in both theory and numerics.
Demetre Kazaras:The geometry and topology of positive scalar curvature
- Graduate/Faculty Seminar,Uploaded Videos ( 1597 Views )I will give an informal overview of the history and status of my field. Local invariants of Riemannian metrics are called curvature, the weakest of which is known as "scalar curvature." The study of metrics with positive scalar curvature is very rich with >100 year old connections to General Relativity and smooth topology. Does this geometric condition have topological implications? The answer turns out to be "yes," but mathematicians continue to search for the true heart of the positive scalar curvature conditions.
Margaret Regan : Using homotopy continuation to solve parametrized polynomial systems in applications
- Graduate/Faculty Seminar,Uploaded Videos ( 1690 Views )Many problems that arise in mathematics, science, and engineering can be formulated as solving a parameterized system of polynomial equations which must be solved for given instances of the parameters. One way to solve these systems is to use a common technique within numerical algebraic geometry called homotopy continuation. My talk will start with background on homotopy continuation and parametrized polynomial systems, followed by applications to problems in computer vision and kinematics. Of these, I will first present a new approach which uses locally adaptive methods and sparse matrix calculations to solve parameterized overdetermined systems in projective space. Examples will be provided in 2D image reconstruction to compare the new methods with traditional approaches in numerical algebraic geometry. Second, I will discuss a new definition of monodromy action over the real numbers which encodes tiered characteristics regarding real solutions. Examples will be given to show the benefits of this definition over a naive extension of the monodromy group (over the complex numbers). In addition, an application in kinematics will be discussed to highlight the computational method and impact on calibration.
Liz Munch : Failure Filtrations and Coverage of Fenced Sensor Networks: An Application of Computational Topology
- Graduate/Faculty Seminar ( 134 Views )Although originally formed as an esoteric field of study, in the last few decades Algebraic Topology has emerged as a vastly applicable field. In this talk we will discuss the basics of Computational Topology and an application to one such coverage problem in sensor networks which even involves a little probability. This talk will be accessible to anyone who enjoys doing math via lots and lots of pictures.
Shrawan Kumar : Topology of Lie groups
- Graduate/Faculty Seminar ( 171 Views )I will give an overview of some of the classical results on the topology of Lie groups, including Hopf's theorem which fully determines the cohomology algebra over the real numbers of any Lie group. We will also discuss how the deRham cohomology of a compact Lie group can be represented by bi-invariant forms. In addition, we will discuss first and the second homotopy groups of Lie groups.
Paul Aspinwall : The Ubiquity of the ADE Classification
- Graduate/Faculty Seminar ( 250 Views )Many classes of mathematical objects turn out to be classified in the same way --- two infinite series and 3 "exceptional" objects. These include symmetries of 3-dimensional solids, rigid singularities, certain types of Lie algebras, positive definite even intersection forms, etc. Discovering why such classes should have the same classification has led to many beautiful ideas and observations. I will give a review of some of the basic ideas (assuming very little in the way of prerequisites) and I may have time to say why string theory has been important in this context.
Zhennan Zhou : Semi-classical Schrodinger equation in the electromagnetic field: approximations and numerics
- Graduate/Faculty Seminar ( 210 Views )I will discuss the semi-classical Schrodinger equation with vector potentials, and its challenges in analysis and in numerical simulations. The time splitting spectral method method will be introduced to solve the equation directly, which is believed to have the optimal mesh strategy. Afterwards. a series of wave packet based approximation approaches will be introduced, like the Gaussian beam method, Hagedorn wave packets method and the Gaussian wave packet transformation method.
Siming He : Suppression of Chemotactic blow-up through fluid flows
- Graduate/Faculty Seminar ( 189 Views )The Patlak-Keller-Segel equations (PKS) are widely applied to model the chemotaxis phenomena in biology. It is well-known that if the total mass of the initial cell density is large enough, the PKS equations exhibit finite time blow-up. In this talk, I will present some recent results on applying additional fluid flows to suppress chemotactic blow-up in the PKS equations.
Paul Bendich : Topological Data Analysis
- Graduate/Faculty Seminar ( 135 Views )TDA is now about fifteen years old, and is quickly becoming a widely applied tool in data analysis. In this talk, I'll describe how homology groups, a traditional algebraic invariant, can be turned into persistence diagrams, a robust statistical tool for dealing with high-dimensional data or embedded geometric objects. Time permitting, this talk should have some theory, some applications, and some algorithms, and perhaps even a proof.
Ioannis Sgouralis : Renal autoregulation in a dynamic nephrovascular model
- Graduate/Faculty Seminar ( 124 Views )Renal autoregulation stabilizes kidney functions and provides protection against blood pressure fluctuations. Autoregulation is mediated by two mechanisms: the *myogenic response,* where increased blood pressure elicits vascular constriction, and *tubuloglomerular feedback,* where salt excretion is balanced by adjustments of filtration rate. Coupling of the two mechanisms give rise to complex behaviours that are challenging to analyse. In the talk, I will describe a mathematical model of renal autoregulation, which represents both mechanisms and thus can be used to study the interactions developed among them. I will provide the necessary physiological background, and I will focus on the mathematical formulation of the involved processes. The talk will be accessible to everyone with basic understanding of differential equations.
Christopher O'Neill : Mesoprimary Decomposition of Binomial Ideals using Monoid Congruences
- Graduate/Faculty Seminar ( 134 Views )Many algebraic objects are notorious for being easy to define, but hard to find explicitly. However, certain algebraic objects, when viewed with the "correct" combinatorial framework, become much easier to actually find. This allows us to compute much larger examples by hand, and often gives us insight into the object's underlying structure. In this talk, we will define irreducible decompositions of ideals, and explore their underlying combinatorial structure in the special case of monomial ideals in polynomial rings. As time permits, we will look at recent results in the case of binomial ideals. This talk will be accessible to anyone who has taken a course in Abstract Algebra.
Kash Balachandran : The Kakeya Conjecture
- Graduate/Faculty Seminar ( 134 Views )In 1917, Soichi Kakeya posed the question: What is the smallest amount of area required to continuously rotate a unit line segment in the plane by a full rotation? Inpsired by this, what is the smallest measure of a set in $\mathbb{R}^n$ that contains a unit line segment in every direction? Such sets are called Kakeya sets, and can be shown to have arbitrarily small measure w.r.t. n-dimensional Lebesgue measure [and in fact, measure zero]. The Kakeya conjecture asserts that the Hausdorff and Minkowski dimension of these sets in $\mathbb{R}^n$ is $n$. In this talk, I will introduce at a very elementary level the machinery necessary to understand what the Kakeya conjecture is asking, and how the Kakeya conjecture has consequences for fields diverse as multidimensional Fourier summation, wave equations, Dirichlet series in analytic number theory, and random number generation. I'll also touch on how tools from various mathematical disciplines from additive combinatorics and algebraic geometry to multiscale analysis and heat flow can be used to obtain partial results to this problem. The talk will be geared towards a general audience.
Erin Beckman : A Look at Branching Processes
- Graduate/Faculty Seminar ( 124 Views )In 1873, a man named Francis Galton posed a question in Educational Times, calling for the mathematical study of the extinction of family surnames over time. Within a year, mathematician Henry Watson replied with a solution. But instead of ending there, this question opened up a new direction of mathematics: the study of branching processes. A branching process is a particle system in which the particles undergo splitting or branching events dictated by particular rules. This talk will introduce some examples of these systems (from the basic Galton-Watson model to more general branching-selection models), interesting questions people ask about branching processes, and some recent research done in this area.
Dave Rose : The EilenbergMazur swindle
- Graduate/Faculty Seminar ( 137 Views )At some point in every mathematician's life they have seen the paradoxical 'proof' that 1=0 obtained by different groupings of the infinite sum 1-1+1-1+... As we learn, the issue is that this series does not converge. The Eilenberg-Mazur swindle is a twist on this argument which shows that A+B+A+B+... = 0 implies that A=0=B in certain situations where we can make sense of the infinite sum. In this talk, we will explore these swindles, touching on many interesting areas of mathematics along the way.
Gero Friesecke : Twisted X-rays and the determination of atomic structure
- Graduate/Faculty Seminar ( 116 Views )We find exact solutions of Maxwell's equations which yield discrete Bragg-peak-type diffraction patterns for helical structures, in the same way in which plane waves yield discrete diffraction patterns of crystals. We call these waves 'twisted X-rays', on account of its 'twisted' waveform. As in the crystal case, the atomic structure can be determined from the diffraction pattern. We demonstrate this by recovering the structure of the Pf1 virus (Protein Data Bank entry 1pfi) from its simulated diffraction data under twisted X-rays.
The twisted waves are found in a systematic way, by first answering a simpler question: could we derive plane waves from the goal that the diffraction pattern crystals is discrete? The answer is yes. Constructive interference at the intensity maxima trivially comes from the fact that the waves share the discrete translation symmetry of crystals. Destructive interference off the maxima is much more subtle, and - as I will explain in the talk - can be traced to the fact that the waves have a larger, continuous translation symmetry. Replacing the continuous translation group by the continuous helical group which extends the discrete symmetry of helical structures leads to twisted waves.
Once the waveforms are found, discreteness (or mathematically, extreme sparsity) of the diffraction pattern of helices under these waves can be proven by appealing to the generalisation of the Poisson summation formula to abelian groups which goes back to A. Weil, whose motivation came from number theory rather than structural biology.
Joint work with Dominik Juestel (TUM) and Richard James (University of Minnesota), SIAM J. Appl. Math. 76 (3), 2016, and Acta Cryst. A72, 190, 2016.
Dmitry Vagner : Higher Dimensional Algebra in Topology
- Graduate/Faculty Seminar ( 219 Views )In his letter, "Pursuing Stacks," Grothendieck advocated to Quillen for the use of "higher" categories to encode the higher homotopy of spaces. In particular, Grothendieck dreamt of realizing homotopy n-types as n-groupoids. This powerful idea both opened the field of higher dimensional algebra but also informed a paradigm in which the distinction between topology and algebra is blurred. Since then, work by Baez and Dolan among others further surveyed the landscape of higher categories and their relationship to topology. In this talk, we will explore this story, beginning with some definitions and examples of higher categories. We will then proceed to explain "the periodic table of higher categories" and the four central hypotheses of higher category theory. In particular, these give purely algebraic characterizations of homotopy types, manifolds, and generalized knots; and account for the general phenomena of stabilization in topology. No prerequisites beyond basic ideas in algebraic topology will be expected.
 yes
yes























