Yossi Farjoun : Solving Conservation Law and Balance Equations by Particle Management
- Applied Math and Analysis ( 101 Views )Conservation equations are at the heart of many interesting and important problems. Examples come from physics, chemistry, biology, traffic and many more. Analytically, hyperbolic equations have a beautiful structure due to the existence of characteristics. These provide the possibility of transforming a conservation PDE into a system of ODE and thus greatly reducing the computational effort required to solve such problems. However, even in one dimension, one encounters problems after a short time.
The most obvious difficulty that needs to be dealt with has to do with the creation of shocks, or in other words, the crossing of characteristics. With a particle based method one would like to avoid a situation when one particle overtakes a neighboring one. However, since shocks are inherent to many hyperbolic equations and relevant to the problems that one would like to solve, it would be good not to ``smooth away'' the shock but rather find a good representation of it and a good solution for the offending particles.
In this talk I will present a new particle based method for solving (one dimensional, scalar) conservation law equations. The guiding principle of the method is the conservative property of the underlying equation. The basic method is conservative, entropy decreasing, variation diminishing and exact away from shocks. A recent extension allows solving equations with a source term, and also provides ``exact'' solutions to the PDE. The method compares favorably to other benchmark solvers, for example CLAWPACK, and requires less computation power to reach the same resolution. A few examples will be shown to illustrate the method, with its various extensions. Due to the current limitation to 1D scalar, the main application we are looking at is traffic flow on a large network. Though we still hope to manage to extend the method to either systems or higher dimensions (each of these extensions has its own set of difficulties), I would be happy to discuss further possible applications or suggestions for extensions.
Jason Mireles-James : Adaptive Set-Oriented Algorithms for Conservative Systems
- Presentations ( 131 Views )We describe an automatic chaos verification scheme based on set oriented numerical methods, which is especially well suited to the study of area and volume preserving diffeomorphisms. The novel feature of the scheme is an iterative algorithm for approximating connecting orbits between collections of hyperbolic fixed and periodic points with greater and greater accuracy. The algorithm is geometric rather than graph theoretic in nature and, unlike existing methods, does not require the computation of chain recurrent sets. We give several example computations in dimension two and three.
William Allard : Currents in metric spaces
- Geometry and Topology ( 96 Views )Motivated by the need to formulate and solve Plateau type problems in higher dimensions and codimensions, normal and integral currents were introduced by Federer and Fleming around 1960; their work was, to some extent a generalization of earlier work by DeGeorgi in codimension one as well as the work of Reifenberg in arbitrary codimensions. Since then a great deal of work has been done on the Plateau problem and related variational problems. This work has always been based on geometric measure theory. The so-called closure theorem for integral currents and the boundary rectifiability theorem are essential ingredients in all of this work; these theorems depend on the Besicovitch-Federer structure theory for set of finite Hausdorff measure in Euclidean space. More recently, in the work of Ambrosio and others, a useful theory of Sobolev spaces for functions with values in an arbitrary metric space has been developed and applied to a variety of problems. Ambrosio and Kirchheim have developed a theory of currents in metric spaces in which they are able to give geometrically appealing proofs of generalizations of the aforementioned closure and rectifiability theorems using some ideas of Almgren and DeGiorgi and avoiding the use of the Besicovitch-Federer structure theory. In this talk I will describe how they do it.
Daniel Gauthier : Nonlinear stability analysis of a time-delay opto-electronic oscillator
- Nonlinear and Complex Systems ( 160 Views )I will describe some recent work on the dynamics of an optoelectronic time-delay oscillator that displays high-speed chaotic behavior with a flat, broad power spectrum. The chaotic state coexists with a linearly stable fixed point, which, when subjected to a finite-amplitude perturbation, loses stability initially via a periodic train of ultrafast pulses. A nonlinear stability analysis is required to understand the device dynamics. Through such an analysis, an approximate mapping is derived that does an excellent job of capturing the observed instability. The oscillator provides a simple device for fundamental studies of time-delay dynamical systems and can be used as a building block for ultrawide-band sensor networks. The results of this study recently appeared in print and can be found here: PRL The work is the part of Kristine Callan's PhD dissertation research and was in collaboration with Zheng Gao, Lucas Illing, and Eckehard Schoell.
Wenjun Ying : A Fast Accurate Boundary Integral Method for the Laplace Equation
- Applied Math and Analysis ( 168 Views )Boundary value and interface problems for the Laplace equation are often solved by boundary integral methods due to the reduction of dimensionality and its flexibility in domain geometry. However, there are two well-known computational issues with the boundary integral method: (a) evaluation of boundary integrals at points close to domain boundaries usually has low order accuracy; (b) the method typically yields dense coefficient matrices in the resulting discrete systems, which makes the matrix vector multiplication very expensive when the size of the system is very large. In this talk, I will describe a fast accurate boundary integral method for the Laplace boundary value and interface problems. The algorithm uses the high order accurate method proposed by (Beale and Lai 2001) for evaluation of the boundary integrals and applies the fast multipole method for the dense matrix vector multiplication. Numerical results demonstrating the efficiency and accuracy of the method will be presented.
Jake Bouvrie : Learning and Synchronization in Stochastic Neural Ensembles
- Graduate/Faculty Seminar ( 97 Views )We consider a biological learning model composed of coupled stochastic neural ensembles obeying a nonlinear gradient dynamics. The dynamics optimize a simple error criterion involving noisy observations provided by the environment, leading to a function that can be used to make decisions in the future. The uncertainty of the resulting decision function is characterized, and shown to be controlled in large part by trading off coupling strength (and/or network topology) against the ambient neuronal noise. Further connections with classical regularization notions in statistical learning theory will also be explored.
Sergey Cherkis : Moduli Spaces of Yang-Mills Instantons on multi-Taub-NUT Spaces
- Geometry and Topology ( 110 Views )We formulate the Nahm transform producing self-dual Hermitian connections on Asymptotically Locally Flat hyperkaehler manifolds. Using this formulation we describe the moduli spaces of such connections and explicitly compute their asymptotic metrics.
Thomas Peacock : Sailing on Diffusion
- Nonlinear and Complex Systems ( 111 Views )Buoyancy-driven flows, which are fluid flows driven by spatial variations of fluid density, play many key roles in the environment. Examples include winds in valleys and over glaciers, mineral transport in rock fissures, and ocean boundary mixing. To date, however, all investigations of buoyancy-driven flow have considered flow induced by a fixed boundary that influences fluid density (e.g. by heating or cooling). We have discovered that buoyancy-driven flows provide a previously unrecognized means of propulsion for freely-floating objects, and we demonstrate this new concept to surprising effect in a series of laboratory experiments.
Jim Nolen : On the great effect of small noise
- Graduate/Faculty Seminar ( 107 Views )This talk will include some PDE, some probability, and some asymptotic analysis. The FKPP equation is a nonlinear partial differential equation that admits traveling wave solutions. It has been used as a simple model for many phenomena involving a stable state invading an unstable state (for example, an advantageous gene spreading through a population). Experiments and numerical simulations show that the speed at which the wave moves is much slower than what is predicted by the deterministic, continuum equation. One way to resolve this discrepancy is to account for the role of noise in the model by adding a stochastic term in the equation (i.e. a stochastic partial differential equation). Analysis of the SPDE has shown that very small noise in the equation introduces a very large correction to the speed associated with the deterministic model. I will explain the basics of the deterministic and stochastic equations, and I will explain some ideas about the asymptotic analysis of the stochastic waves. I hope to have time to explain some open problems related to this topic.
Patricia Hersh : Topology and combinatorics of regular CW complexes
- Algebraic Geometry ( 148 Views )Anders Björner characterized which finite, graded partially ordered sets (posets) are closure posets of finite, regular CW complexes, and he also observed that a finite, regular CW complex is homeomorphic to the order complex of its closure poset. One might therefore hope to use combinatorics to determine topological structure of stratified spaces by studying their closure posets; however, it is possible for two different CW complexes with very different topological structure to have the same closure poset if one of them is not regular. I will talk about a new criterion for determining whether a finite CW complex is regular (with respect to a choice of characteristic functions); this will involve a mixture of combinatorics and topology. Along the way, I will review the notions from topology and combinatorics we will need. Finally I will discuss an application: the proof of a conjecture of Fomin and Shapiro, a special case of which says that the Schubert cell decomposition of the totally nonnegative part of the space of upper triangular matrices with 1's on the diagonal is a regular CW complex homeomorphic to a ball.
Natasa Sesum : On the extension of the mean curvature flow and the Ricci flow
- Geometry and Topology ( 106 Views )In the talk we will discuss curvature conditions under which we can guarantee the existence of a smooth solution to the Ricci flow and the mean curvature flow equation. These are improvements of Hamilton's and Husiken's results on extending the Ricci flow and the mean curvature flow, under conditions that the norm of Riemannian curvature and the norm of the second fundamental form are uniformly bounded along the flow in finite time, respectively.
Thomas Witelski : Mean field models and transient effects for coarsening dynamics in fluid films
- Nonlinear and Complex Systems ( 104 Views )Motivated by the dewetting of viscous thin films on hydrophobic substrates, we study models for the coarsening dynamics of interacting localized structures in one dimension. For the thin films problem, lubrication theory yields a Cahn-Hilliard-type governing PDE which describes spinodal dewetting and the subsequent formation of arrays of metastable fluid droplets. The evolution for the masses and positions of the droplets can be reduced to a coarsening dynamical system (CDS) consisting of a set of coupled ODEs and deletion rules. Previous studies have established that the number of drops will follow a statistical scaling law, N(t)=O(t^{-2/5}). We derive a Lifshitz-Slyozov-Wagner-type (LSW) continuous model for the drop size distribution and compare it with discrete models derived from the CDS. Large deviations from self-similar LSW dynamics are examined on short- to moderate-times and are shown to conform to bounds given by Kohn and Otto. Insight can be applied to similar models in image processing and other problems in materials science. Joint work with M.B. Gratton (Northwestern Applied Math).
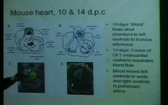
Laura Miller : Scaling effects in heart development: Changes in bulk flow patterns and the resulting forces
- Applied Math and Analysis ( 92 Views )When the heart tube first forms, the Reynolds number describing intracardial flow is only about 0.02. During development, the Reynolds number increases to roughly 1000. The heart continues to beat and drive the fluid during its entire development, despite significant changes in fluid dynamics. Early in development, the atrium and ventricle bulge out from the heart tube, and valves begin to form through the expansion of the endocardial cushions. As a result of changes in geometry, conduction velocities, and material properties of the heart wall, the fluid dynamics and resulting spatial patterns of shear stress and transmural pressure change dramatically. Recent work suggests that these transitions are significant because fluid forces acting on the cardiac walls, as well as the activity of myocardial cells which drive the flow, are necessary for correct chamber and valve morphogenesis.
In this presentation, computational fluid dynamics was used to explore how spatial distributions of the normal forces and shear stresses acting on the heart wall change as the endocardial cushions grow, as the Reynolds number increases, and as the cardiac wall increases in stiffness. The immersed boundary method was used to simulate the fluid-structure interaction between the cardiac wall and the blood in a simplified model of a two-dimensional heart. Numerical results are validated against simplified physical models. We find that the presence of chamber vortices is highly dependent upon cardiac cushion height and Reynolds number. Increasing cushion height also drastically increases the shear stress acting on the cushions and the normal forces acting on the chamber walls.
Miles Crosskey : Spectral bounds on empirical operators
- Graduate/Faculty Seminar ( 99 Views )Many machine learning algorithms are based upon estimating eigenvalues and eigenfunctions of certain integral operators. In practice, we have only finitely many randomly drawn points. How close are the eigenvalues and eigenfunctions of the finite dimensional matrix we construct in comparison to the infinite dimensional integral operator? In what way can we say these two are close if they do not even operate on the same spaces? To answer these questions, I will be showing some results from a paper "On Learning with Integral Operators" by Rosasco, Belkin, and De Vito.
Leonid Berlyand : Flux norm approach to finite-dimensional homogenization approximation with non-separated scales and high contrast
- Applied Math and Analysis ( 152 Views )PDF Abstract
Classical homogenization theory deals with mathematical models of strongly
inhomogeneous media described by PDEs with rapidly oscillating coefficients
of the form A(x/\epsilon), \epsilon → 0. The goal is to approximate this problem by a
homogenized (simpler) PDE with slowly varying coefficients that do not depend
on the small parameter \epsilon. The original problem has two scales: fine
O(\epsilon) and coarse O(1), whereas the homogenized problem has only a coarse
scale.
The homogenization of PDEs with periodic or ergodic coefficients and
well-separated scales is now well understood. In a joint work with H. Owhadi
(Caltech) we consider the most general case of arbitrary L∞ coefficients,
which may contain infinitely many scales that are not necessarily well-separated.
Specifically, we study scalar and vectorial divergence-form elliptic PDEs with
such coefficients. We establish two finite-dimensional approximations to the
solutions of these problems, which we refer to as finite-dimensional homogenization
approximations. We introduce a flux norm and establish the error
estimate in this norm with an explicit and optimal error constant independent
of the contrast and regularity of the coefficients. A proper generalization of
the notion of cell problems is the key technical issue in our consideration.
The results described above are obtained as an application of the transfer
property as well as a new class of elliptic inequalities which we conjecture.
These inequalities play the same role in our approach as the div-curl lemma
in classical homogenization. These inequalities are closely related to the issue
of H^2 regularity of solutions of elliptic non-divergent PDEs with non smooth
coefficients.
Michael Henry : Connections between existing Legendrian knot invariants
- Geometry and Topology ( 127 Views )In this talk, we will investigate existing Legendrian knot invariants and discuss new connections between the theory of generating families and the Chekanov-Eliashberg differential graded algebra (CE-DGA). The geometric origins of the CE-DGA are Floer theoretic in nature and come out of the Symplectic Field Theory developed by Eliashberg and Hofer. On the other hand, Legendrian invariants derived from the study of 1-parameter families of smooth functions (called generating families) are Morse theoretic in nature. In the last decade, connections have been found between the Legendrian invariants derived using these two methods. In this talk, I will try to provide a clearer picture of the relationship between generating families and the CE-DGA.
Dan Lee : Black hole uniqueness and Penrose inequalities
- Geometry and Topology ( 115 Views )I will tell two stories. The first is the story of static spacetimes with black hole boundaries and the attempt to classify them. The second is the story of the Penrose inequality. I will then weave these two stories together in the setting of negative curvature. This last part is a report on joint work-in-progress with A. Neves.
Hongqiang Wang : Non-equipartition in a binary granular system and measurement of velocity distribution in a 3D vibrated granular system
- Nonlinear and Complex Systems ( 112 Views )Fluidized granular systems with inelastic inter-particle collisions exhibit distinguishing behavior from it's elastic counterpart. Two species of particles in a binary granular system typically do not have the same mean kinetic energy, in contrast to the equipartition of energy required in equilibrium. It is found that not only the mechanical properties of these two types of particles, but also the heating mechanism plays an important role in affecting the extent of nonequipartition of kinetic energy, even in the bulk of the system. An experimental measurement of the velocity distribution of a 3D vibration fluidized granular medium by spatial resolved high speed video particle tracking is also reported. It is found that the distribution is wider than a Gaussian and broadens continuously with increasing volume fraction.
Junping Wang : Mathematics and Computation of Sediment Transport for Open Channels
- Applied Math and Analysis ( 106 Views )The purpose of this presentation is to communicate some mathematical and computational issues in sediment transport for open channels. The main topics are: (1) mathematical simulation for surface and subsurface fluid flow, (2) mathematical modeling of sediment transport in open channels as a 2D problem, and (3) numerical methods for fluid flow and sediment transport.
Graham Cox : Unsolvable problems in geometry and topology
- Graduate/Faculty Seminar ( 113 Views )The resolution of Hilbert's tenth problem yields the following unsolvability result: there is no algorithm for determining whether or not a given polynomial equation p(x_1,...,x_n) = 0 with integer coefficients will admit an integer solution. After a few definitions and examples, I will discuss another well-known unsolvable problem: the word problem for finitely presented groups. It can be shown that there is no algorithm for determining when an arbitrary word in a finitely presented group is trivial. This has many remarkable topological consequences, including the result that there is no algorithm that will determine when two given manifolds are homeomorphic (provided the dimension is at least four). The unsolvability theorem also has significant geometric applications, allowing one to prove that certain manifolds admit an infinite number of contractible closed geodesics (regardless of the Riemannian structure).
Graeme Wilkin : Morse theory and stable pairs
- Geometry and Topology ( 137 Views )In the early 1980s Atiyah and Bott described a new approach to studying the cohomology of the moduli space of stable bundles: the equivariant Morse theory of the Yang-Mills functional. There are many other interesting moduli spaces that fit into a similar framework, however the catch is that the total space is singular, and it is not obvious how to construct the Morse theory of the appropriate functional. In this talk I will describe how to get around these difficulties for the moduli space of stable pairs, for which we prove a Kirwan surjectivity theorem and give a Morse-theoretic interpretation of the change in cohomology due to a flip. This builds upon earlier work with George Daskalopoulos, Jonathan Weitsman and Richard Wentworth for rank 2 Higgs bundles.
Badal Joshi : A coupled Poisson process model for sleep-wake cycling
- Applied Math and Analysis ( 115 Views )Sleep-wake cycling is an example of switching between discrete states in mammalian brain. Based on the experimental data on the activity of populations of neurons, we develop a mathematical model. The model incorporates several different time scales: firing of action potentials (milliseconds), sleep and wake bout times (seconds), developmental time (days). Bifurcation diagrams in a deterministic dynamical system gives the occupancy time distributions in the corresponding stochastic system. The model correctly predicts that forebrain regions help to stabilize wake state and thus modifies the wake bout distribution.
Aubrey HB : Persistent Homology
- Graduate/Faculty Seminar ( 174 Views )Persistent Homology is an emerging field of Computational Topology that is developing tools to discover the underlying structure in high-dimensional data sets. I will discuss the origins and main concepts involved in Persistent Homology in an accessible way, with illustrations and comprehensive examples. If time allows, I will also describe some current, as well as, future applications of Persistent Homology.
Gábor Székelyhidi : Greatest lower bounds on the RIcci curvature of Fano manifolds
- Geometry and Topology ( 111 Views )On a Fano manifold M we study the supremum of the possible t such that there is a Kähler metric in c_1(M) with Ricci curvature bounded below by t. We relate this to Aubin's continuity method for finding Kähler-Einstein metrics and we give bounds on it for certain manifolds.
Cécile Piret : Overcoming the Gibbs Phenomenon Using a Modified Radial Basis Functions Method
- Applied Math and Analysis ( 122 Views )The Radial Basis Functions (RBF) method is not immune from the disastrous effects of the Gibbs phenomenon. When interpolating or solving PDEs whose solutions are piecewise smooth functions, the RBF method loses its notorious spectral accuracy. In this talk, a new method will be presented, based on the RBF method, which incorporates singularities using Heaviside functions and which keeps track of their location using the level set method. The resulting sharp interface method will be shown to recover the lost spectral accuracy and thus overcome the Gibbs phenomenon altogether.
Benoit Charbonneau : Gauge theory and modern problems in geometry
- Graduate/Faculty Seminar ( 113 Views )I will survey some modern questions in geometry that were solved or that could be solved using tools of gauge theory. This talk should be accessible to first year grad students, and of interest to anyone who is curious about what happens in the field of geometry.

 yes
yes